The Blue Filter Allows Two Wavelengths of the Mercury Spectrum to Pass Why

SPECTRAL FILTERING

Spectral filtering is most commonly used to either select or eliminate information from an paradigm based on the wavelength of the information. This filtering is normally effected by passing the light through a glass or plastic window that has been specially treated to transmit or blot/reflect some wavelengths. Since the lite inbound a sensor volition have a spectral distribution that depends (mainly) on both the illumination source'due south spectral characteristics and the reflectance of the illuminated scene, using a filter to select image regions with known spectral backdrop can help extract the desired information.

Some applications that utilise spectral filtering are:

• identifying places in a scene that have been marked with a fluorescent dye, past using a spectral filter in front end of the camera that allows just light of the wavelength of the fluorescence to pass through the filter.
• identifying a laser-created light pattern in a scene by using a spectral filter filter in front of the camera that simply allows light of the laser wavelength to pass through. This application is discussed more than in the example below.
• eliminating the infrared component from the light entering the camera by a spectral filter that allows simply visible light through. This filtering is oft applied to either eliminate light arising from infrared position sensors, or to sharpen images as the infrared component of illumination has a tendency to internal reflections on solid country sensors, leading to epitome blurring.

In the starting time 2 applications above, the filters were "band-pass" filters that only allowed lite of a specified wavelength to pass through. In the infrared case, the filter used could accept been a "ring-pass" filter set for visible calorie-free, a "band-cease" filter that eliminates all light above the visible wavelengths, or a "band-stop" filter that eliminates only the lite at the specific wavelength of the infrared position sensor.

In the discussion above, the concept of let or eliminate was used a bit too precisely. In reality, it is non possible to permit only a single wavelength; instead, the filter usually allows a range of wavelengths near to the specified wavelength. Part of the skill of creating filters is to command the narrowness of the range of wavelengths, how well the filter transmits at the desired wavelengths, and how trivial change there is in the relative intensities of the transmitted illumination. For example, the filter used in the laser stripe example below is tuned to a wavelength of 633 nm, with a one-half width of 10 nm. That means that any light exterior the range of 623-643 nm is guaranteed to exist reduced by at least l%. The filter also does non reduce the intensity at 633 nm by more than 5%. Both of these backdrop are ideal because the light amplification by stimulated emission of radiation wavelength is 632.viii nm. Filter manufacturers brand filters with the aforementioned pass wavelength as common laser sources.

More than precisely, if the amount of light transmitted by a filter at wavelength is and the input spectrum intensity at wavelength is , and then the intensity of the light transmitted through the filter at wavelength is . This is illustrated pictorially by:

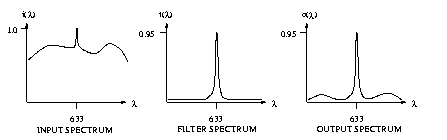
One should be careful to not expect too much from a filter. For instance, suppose that you employ a "cherry" filter to look for carmine objects in a scene. Since ruby-red is a range of wavelengths, the filter will have to take a broad pass band. It is thus likely that it would not profoundly decline other wavelengths of lite. For example, "blue" might be reduced fifty%, merely that however allows a lot of blueish low-cal to pass through the filter. If a blue region were more than than twice equally reflective as a carmine region and the amount of illumination both received were equal at all wavelengths, and so the blue region observed through the ruddy filter might even so be brighter than the red region, but no longer twice equally brilliant. (Note: this case is a trivial imprecise considering the definition of the region and filter colors was not made precise, but the general concept of the statement should be articulate.)

A common use of spectral filtering is in range sensors based on triangulation of a structured light blueprint (eg. a projected lite stripe). The projected low-cal is usually generated using a light amplification by stimulated emission of radiation, because this allows use of a spectral filter that finer transmits only the laser light stripe, irrespective of the other illumination in the scene. While almost typical indoor scene illumination is broad-spectrum and thus has energy at the same wavelength as the light amplification by stimulated emission of radiation, typically the ability at this wavelength is much lower than the laser, and then one tin simply look for the brightest stripe in the prototype. Outdoor scenes are more problematic equally solar illumination is much brighter (e.thou. 100 times) than typical indoor illumination, which may require use of a laser that is more powerful than those that are safety for use with unprotected optics.

This image shows a scene observed by a triangulation sensor, where the brilliant virtually vertical stripe in the center of the image is generated by the laser.

Notice that the stripe is not obviously different from some of the other brilliant features in the scene. If we add a spectral filter to the camera, the image changes to this:

Now the stripe is clearly singled-out and easily extracted from the image. (The image was inverted and slightly contrast enhanced to ameliorate its appearance.) Real range sensors also do consistency checks on the extracted stripes to eliminate other possible sources, such as occasional specular reflections of light sources, which can be much brighter than the diffuse reflected light that is easily eliminated by the spectral filtering.

murphyindes1973.blogspot.com

Source: https://homepages.inf.ed.ac.uk/rbf/CVonline/LOCAL_COPIES/FISHER/specfilt.htm

Share this post